This post deal with the conditions to check whether the roots are real or complex of a cubic equation. A cubic equation always has a real root. The other two roots are real or complex according as the graph cuts the x axis in one or three positions. If the graph touches the x-axis then two roots are equal. If the graph cuts the x-axis in three positions then all the roots are real. If the graph cuts the x-axis in one position then other two roots are complex. When we look at the graph of a general cubic equation we see a cup like structure and a cap like structure. The base of the cup like structure gives local minima and the top of cap like structure gives local maxima. Let us find the positions where the curve bends and changes its direction. We call the cup like structure concave upward and a cap like structure convex upward. The quadratic equation is ax3 + bx2 + cx + d = 0. Let it be equal to y or f(x). y = ax3 + bx2 + cx + d. Now the rate of change of curve is f '(x) = 3ax2 + 2bx + c. f(x) is differentiated to obtain f '(x). We again differentiate f '(x) to obtain f ''(x),
f ''(x) = 6ax + 2b
f ''(x) = 6a(x + b/3a)
Let us check, for what values of x it may give maxima and for what values it may give minima.
There are four cases for it and is given in the following table
When the local maxima and and local minima are on opposite sides of x-axis then all the roots are real.
Let us find the x-coordinate of maxima or minima. It is when f '(x)=0.
Solving 3ax2 + 2bx + c = 0 we get
x = [−2b±√(4b2 − 12ac)]/6a
x = [−b±√(b2 − 3ac)]/3a
or
x1 = [−b+√(b2 − 3ac)]/3a
and
x2 = [−b−√(b2 − 3ac)]/3a Find the value of y for x1 and x2, substituting them in place of x.
For x1 we get y1 = [4b3 − 15abc + 27a2d + (6ac − 2b2)√(b2 −3ac)]/27a2
For x2 we get y2 = [4b3 − 15abc + 27a2d − (6ac − 2b2)√(b2 −3ac)]/27a2
Now local maxima and local minima lie on the opposite sides of the x-axis when y1 and y2 lie on the opposite sides of x-axis. This will happen when they are of opposite sign.
Now four cases arise:
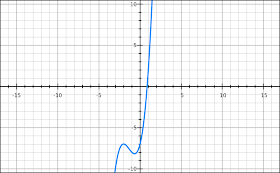
When (b2 − 3ac) is less than zero then y1 and y2 are complex and there is no maxima and minima. Then the equation has only one real root and other two are complex. See the graph for 3x2 + 2x2 + 3x + 4 = 0.
Where (b2 − 3ac) = (4 − 27) < 0.
When y1 or y2 is equal to zero then there are two equal roots.
f ''(x) = 6ax + 2b
f ''(x) = 6a(x + b/3a)
Let us check, for what values of x it may give maxima and for what values it may give minima.
There are four cases for it and is given in the following table
| f ''(x) | x | a | state |
|---|---|---|---|
| > 0 | x < (−b/3a) | a < 0 | minima |
| < 0 | x > (−b/3a) | a < 0 | maxima |
| < 0 | x < (−b/3a) | a > 0 | maxima |
| > 0 | x > (−b/3a) | a > 0 | minima |
When the local maxima and and local minima are on opposite sides of x-axis then all the roots are real.
Let us find the x-coordinate of maxima or minima. It is when f '(x)=0.
Solving 3ax2 + 2bx + c = 0 we get
x = [−b±√(b2 − 3ac)]/3a
or
x1 = [−b+√(b2 − 3ac)]/3a
and
x2 = [−b−√(b2 − 3ac)]/3a
For x1 we get y1 = [4b3 − 15abc + 27a2d + (6ac − 2b2)√(b2 −3ac)]/27a2
For x2 we get y2 = [4b3 − 15abc + 27a2d − (6ac − 2b2)√(b2 −3ac)]/27a2
Now local maxima and local minima lie on the opposite sides of the x-axis when y1 and y2 lie on the opposite sides of x-axis. This will happen when they are of opposite sign.
Now four cases arise:
| y1 | y2 | Remark |
|---|---|---|
| y1< 0 | y2< 0 | One root is real and other two are complex. Graph of y = x3 + 4x2 + 4x − 7 |
| y1< 0 | y2> 0 | All the three roots are real. Graph of y = x3 + 6x2 + 4x − 7 If y1 = 0 or y2 = 0 then there are two equal roots and it may be [−b+√(b2 − 3ac)]/3a or [−b-√(b2 − 3ac)]/3a |
| y1> 0 | y2< 0 | All the three roots are real. Graph of y = − x3 + 4x2 + 4x − 7 If y1 = 0 or y2 = 0 then there are two equal roots. and it may be [−b+√(b2 − 3ac)]/3a or [−b-√(b2 − 3ac)]/3a |
| y1> 0 | y2> 0 | One root is real and other two are complex. Graph of y = x3 + 4x2 + 4x + 7 |
When (b2 − 3ac) is less than zero then y1 and y2 are complex and there is no maxima and minima. Then the equation has only one real root and other two are complex. See the graph for 3x2 + 2x2 + 3x + 4 = 0.
Where (b2 − 3ac) = (4 − 27) < 0.
When y1 or y2 is equal to zero then there are two equal roots.




No comments:
Post a Comment